Pythagorean geometry in Vedic-era texts, centuries before Pythagoras

A Position paper by the Karnataka government on the National Education Policy (NEP) 2020 has revived discussion that what we call the Pythagoras theorem was already known to Indians from the Vedic times.

Historical Background

• The Pythagoras theorem describes the relationship connecting the three sides of a right triangle (one in which one of the angles is 90°).
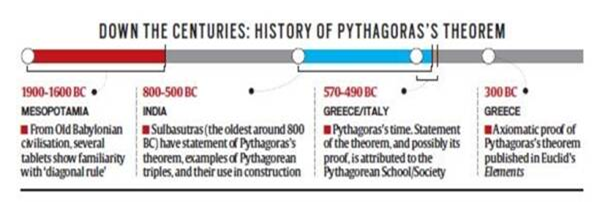
• There are similar references in the sulbasutras, which are texts pertaining to fire rituals (yajnas) performed by Vedic Indians. The oldest of these is the ‘Baudhayana Sulbasutra’.
• Baudhayana Sulbasutra contains a statement of what is called Pythagoras theorem.
• The earliest evidence of a proof comes from a period after the sulbasutras.
• The oldest surviving axiomatic proof of the theorem is in the Elements of Euclid from around 300 BCE.
• It was mentioned in a paper describing geometry in the sulbasutras in ‘Studies in History of Mathematics, Proceedings of Chennai Seminar’ in

What is the evidence that Sulbasutra contains?

• In the first chapter in the Baudhayana Sulbasutra contains, the (areas of the squares) produced separately by the length and the breadth of a rectangle together equal the area (of the square) produced by the diagonal.
• This is observed in rectangles having sides 3 and 4, 12 and 5, 15 and 8, 7 and 24, 12 and 35, 15 and 36.
• Uses:
• The yajna rituals involved construction of altars (vedi) and fireplaces (agni) in a variety of shapes such as isosceles triangles, symmetric trapezia, and rectangles.
• The sulbasutras describe steps towards construction of these figures with prescribed sizes.
  
  

What Pythagoras theorem says?

• The Pythagoras theorem describes the relationship connecting the three sides of a right triangle (one in which one of the angles is 90°): a² + b² = c², where a and b are the two perpendicular sides, and c is the length of the diagonal side.
• If any two sides of a right triangle are known, the theorem allows you to calculate the third side.
• Extended to the sides of squares and rectangles and their diagonals, the equation is of immense importance in construction, navigation and astronomy.

What is the similarity between Sulbasutra’s equation and Pythagoras?

• The Pythagorean equation comes into play in these procedures, which involve drawing perpendiculars.
• These perpendiculars were based on triangles whose sides were in the ratio 3:4:5 or 5:12:13.
• These sides follow the Pythagorean relation, because 3² + 4² = 5², and 5² + 12² = 13². Such combinations are called Pythagorean triples.

Did Indian mathematicians prove the equation?

• The idea of a mathematical proof based on an axiomatic structure is unique to the Greeks.
• Thus in respect of the other cultures, ‘proof’ of a geometrical statement only meant some means of various cultures like one India has in its Vedic times.